Conference: 2nd Summer Math Summer Workshop: Moduli, Simons Institute, Stony Brook, July 2024
Minicourse: Mirror symmetry and big algebras
After surveying some mirror symmetry phenomena in the geometry of Langlands dual
Hitchin systems, we will model the Hitchin system on cominuscule upward flows by the equivariant
cohomology of cominuscule flag varieties like the Grassmannian. Then we will explain how mirror
symmetry implies a synthetic BNR correspondence. To generalise to the non-minuscule case we
will construct a big commutative subalgebra of the Kirillov algebra to model the mirror of the
universal principal bundle in an irreducible representation. We will discuss various aspects of big
algebras such as their geometric interpretation in terms of equivariant cohomology of affine
Schubert varieties, their symmetries, their anatomy, including their skeletons, multiplicity algebras,
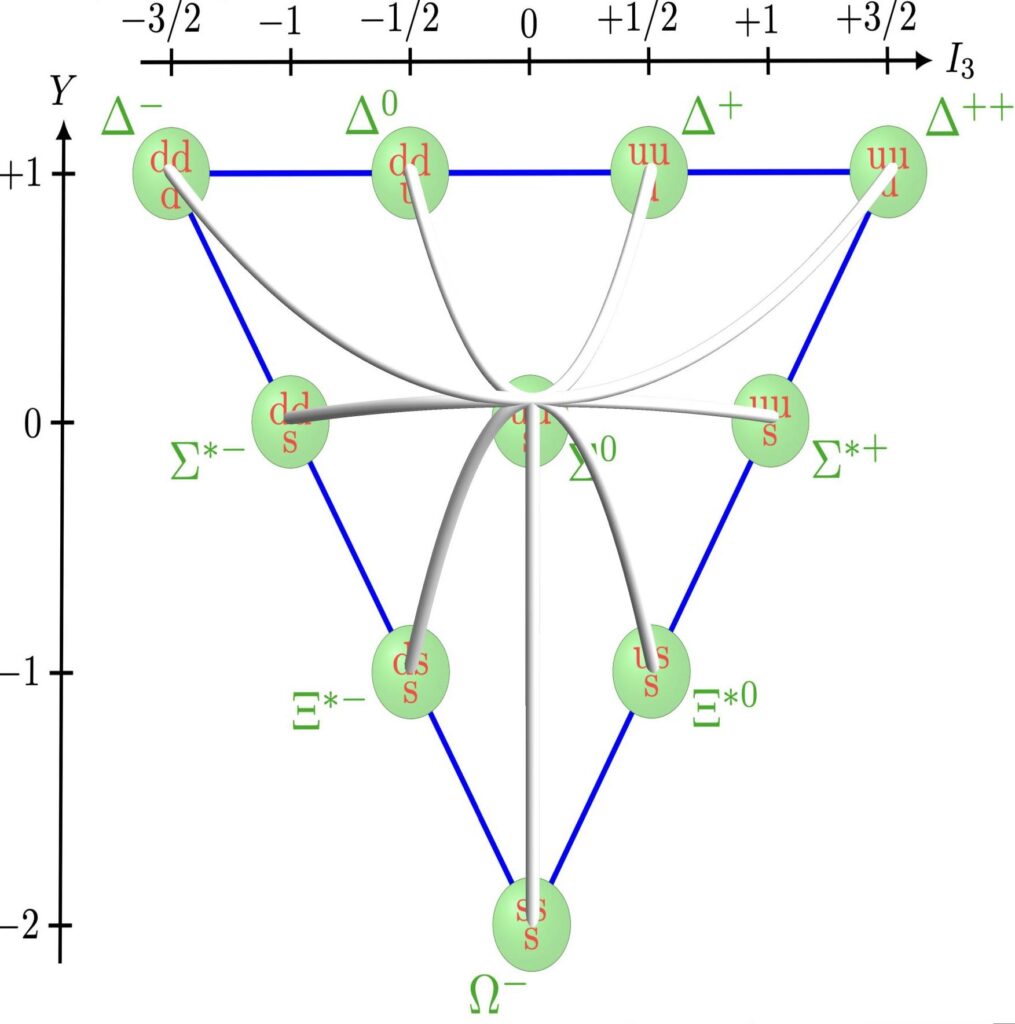
nerves and crystals. We will visualize some small examples related to baryon multiplet.
slides, Lecture 1. Video, Lecture 2. Video, Lecture 3. Video
skeleton over baryon decuplet: